Design summary

Frame construction is planned to be made of aluminium profiles, each parts riveted together. Shell – lifting gas container is 800g sounding balloon.

With hull length    and main diameter    gives fineness ratio  .

Shell membrane stress

Calculation is based on Laplace equation for thin membrane

describing two-dimensional membrane stress with rotational symmetry.

 

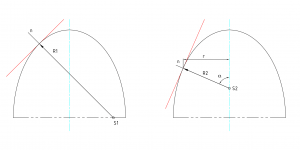
First main radius is given and second main radius can be written as

For given  can be solved  angle of tangential tension as

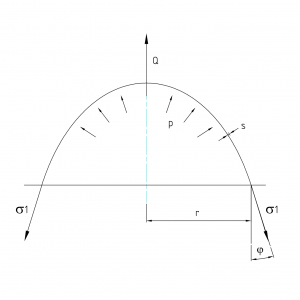
static equilibrium on shell element

where axial force on shell section is

after modifying can be written axial tension

 

substitution back to Laplace eq. will give second tangent tension

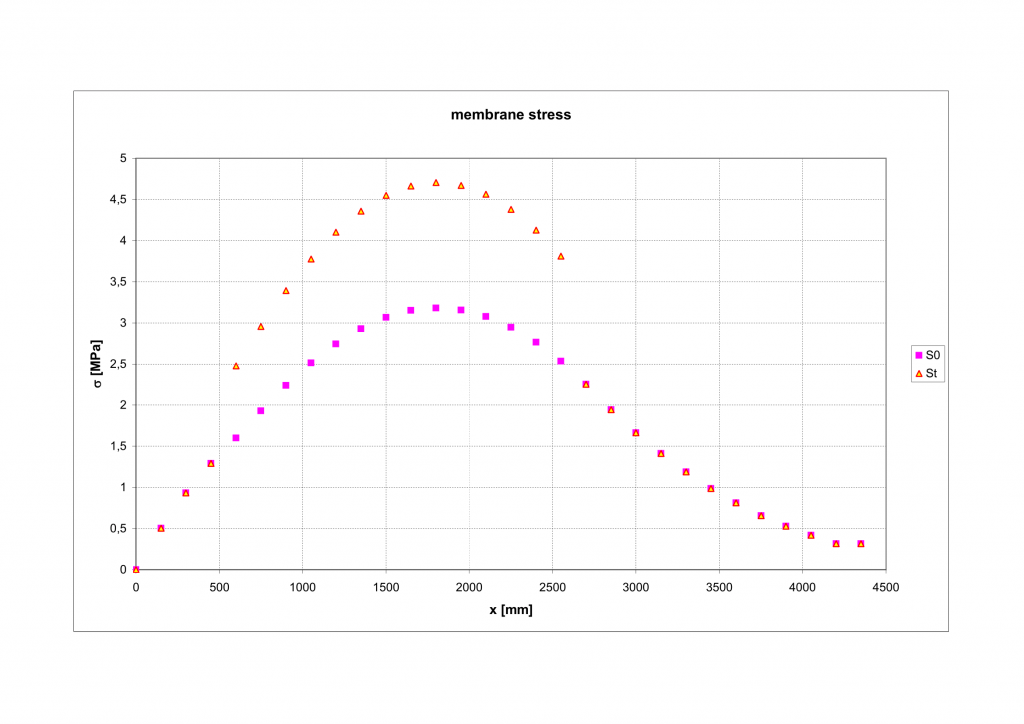
Shell inflating

Graphs computed for inflation of sphere , variables are functions of relative membrane deformation

Internal pressure function  described with equations @ http://journals.sagepub.com/doi/abs/10.1177/108128650200700506

 

balloon parameters: weight 800g

uninflated dia

burst dia 

membrane initial thickness 

critical deformation

membrane thickness depends on deformation by equation

 

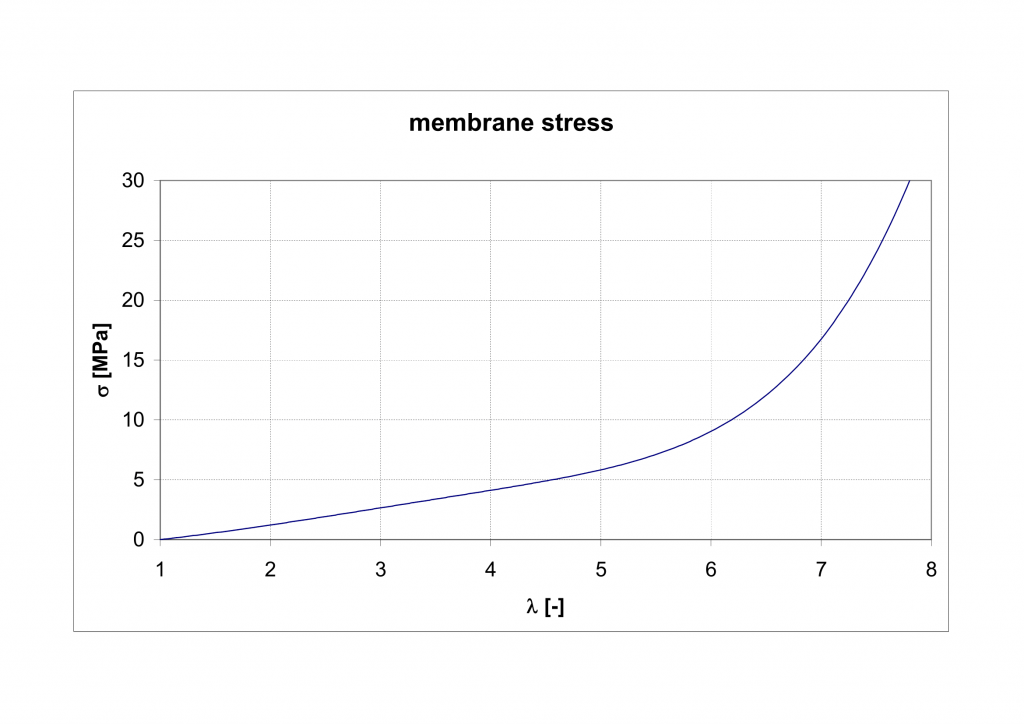
Function of membrane stress and thickness is based on Yeoh model computed by http://www.lajss.org/index.php/LAJSS/article/view/2622

On the basis of experimental test data of sounding balloon manufacturer –  https://novalynx.com/store/pc/400-8200-Series-Weather-Balloons-p882.htm , can be determined, that membrane burst with stress load  for critical deformation . Computed maximal stress of shell is and therefore the membrane load stays in safety area with save coefficient .

Buoyancy

constants:

air density        NTP – normal  temperature and pressure defined as 20^oC and 1 atm

hydrogen density    NTP

shell volume

therefore buoyancy force is 

for fully inflated shell is gross lift force 

 

For designed total weight of airship  , gives free lift of  . To obtain vertical force stability, it is needed to adjust  up to weight equal to gross lift by the ballast. Ballast is situated in front section of gondola. Weight of ballast  will be adjusted in dependency of real airship weight and weather condition (atmospheric pressure and ambient temperature). To adjust position of center of gravity towards to the same vertical line with buoyancy center, which is indispensable for horizontal flight stability, gondola is movable mounted on bottom beam, so the position of COG can be changed with position of gondola.

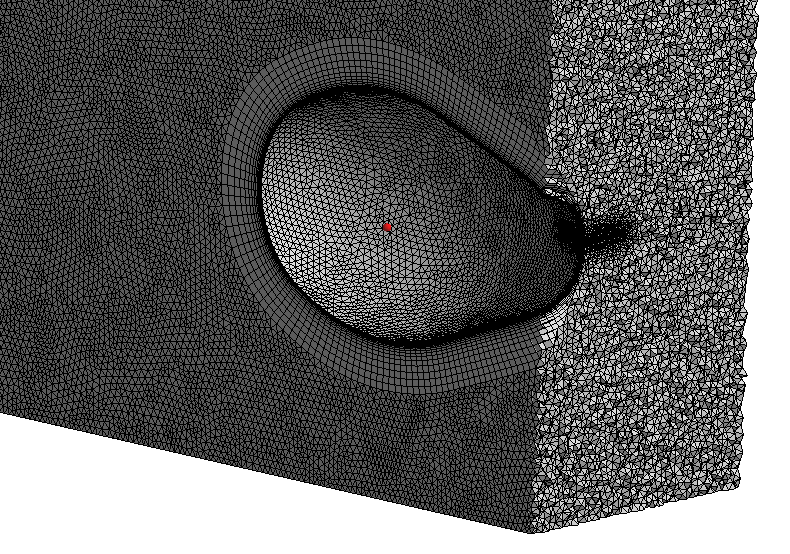
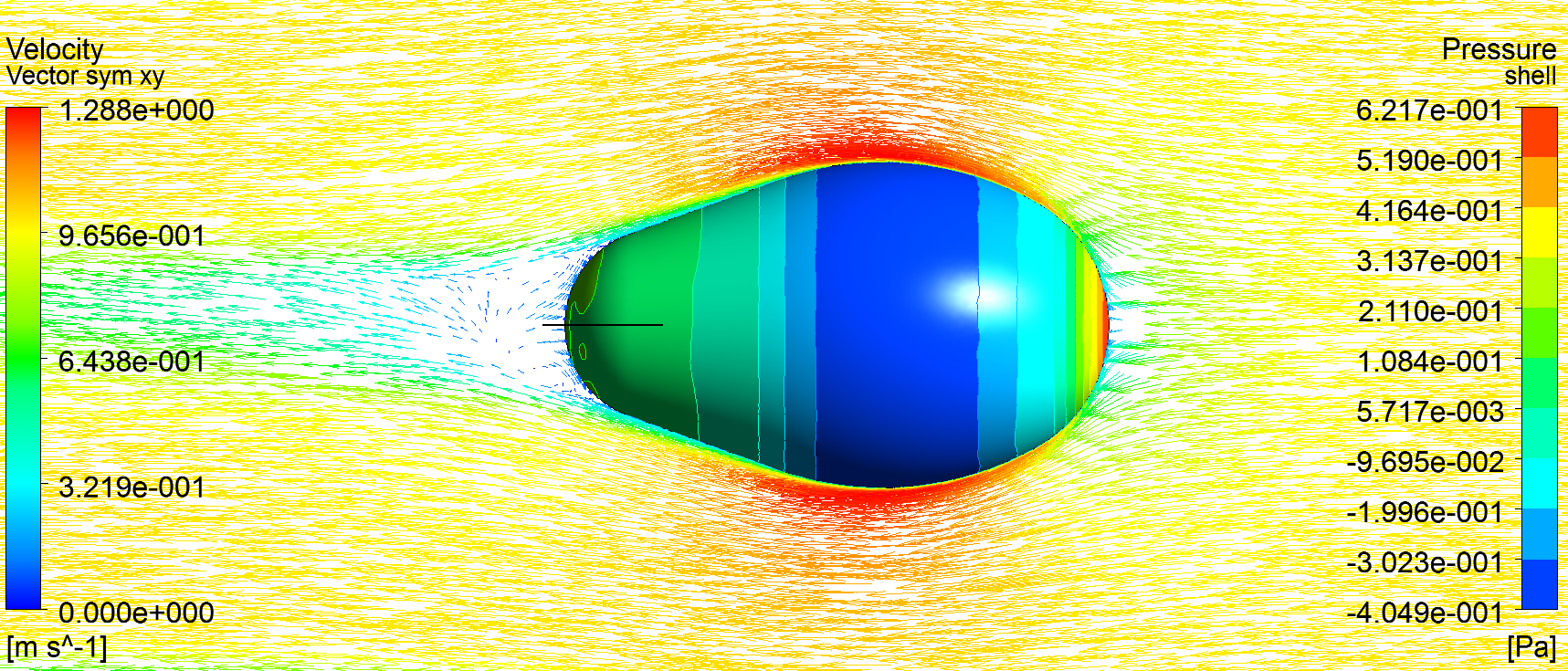
Fluid dynamics

static flow field over shell geometry

 analysis has been solved for travel speed

Reynolds number   , for

main diameter , kinematic viscosity of air 

is

computed shell drag force 

Results for variable travel speed could be obtained with drag coefficient making use of equation

which gives for defined geometry .

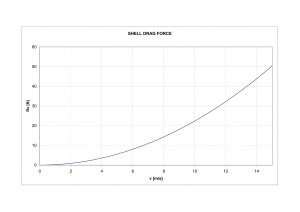
With computed characteristic of dependency shell drag force and travel speed, could be determined the motors thrust force which must be  .

 

 

 

 

 

 

 

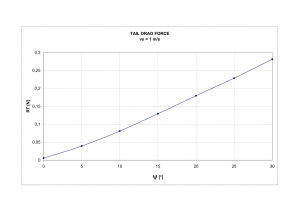
With the same method, tail drag force is computed. Function of rudder acting angle  and tail drag force  is almost linear.

 

 

 

 

 

 

 

 

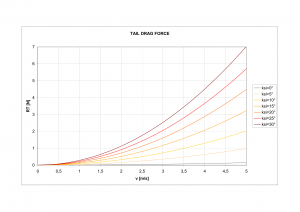
Applying the same formula for drag coefficient, it is computed the exponential characteristic for several rudder angles increasing with square of velocity.

 

 

 

 

 

 

Stability

In horizontal direct flight, center of buoyancy and center of gravity must lie on the same vertical line. This condition is achieved with ballast position adjustment during pre-flight preparation.

On figure, airship drawn in inclined flight, acting forces are shown below.

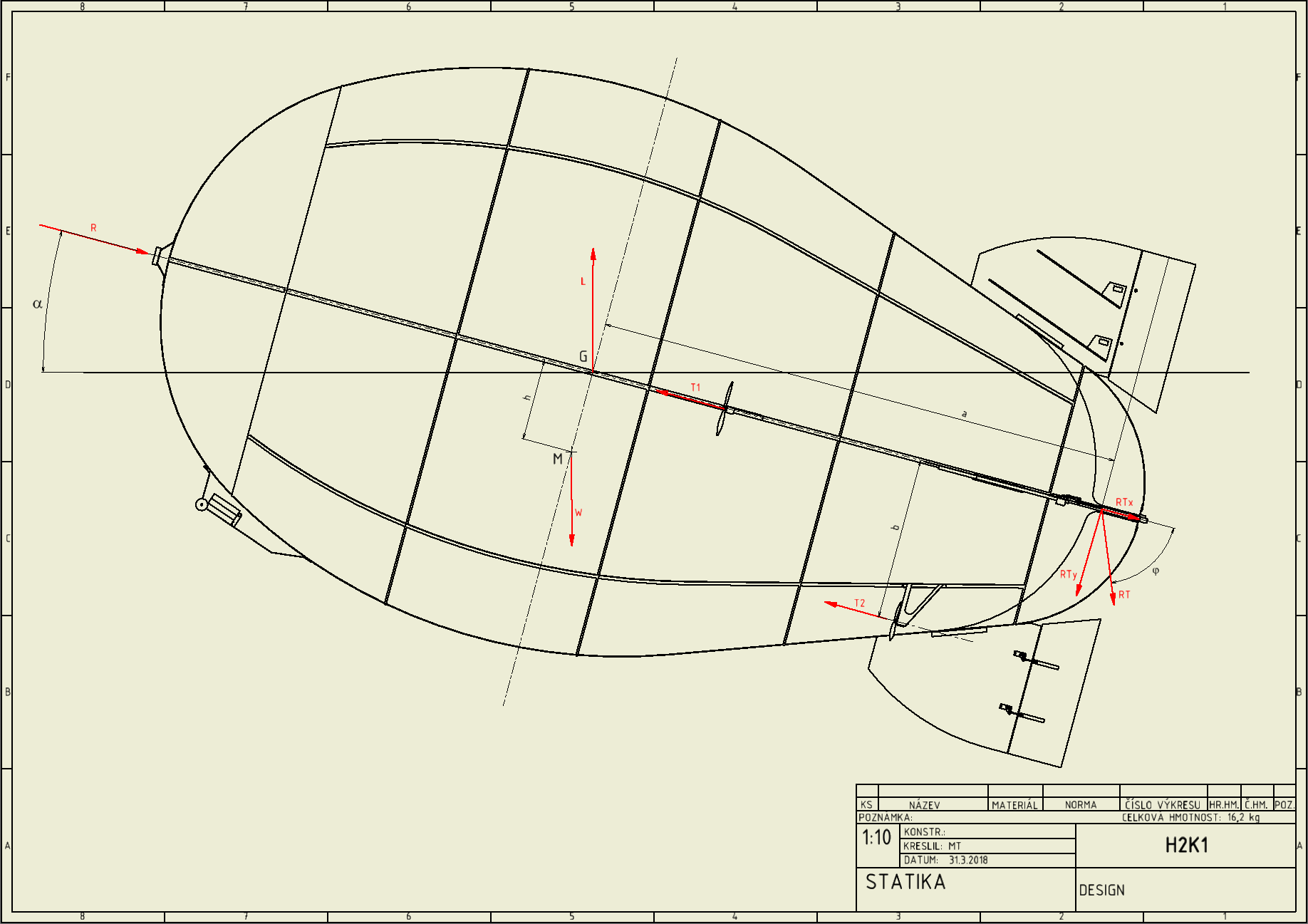
For condition of static equilibrium, can be written for moment about G

Airship thrust engines are designed in four units acting in two parallel couples. Resultant force lies on hull center line and acting directly against drag force . The second one acting in distance  bellow the hull centerline.

During the horizontal flight, most thrust power will be generated with , will be used as compensator or in cooperation with rudder for increasing a total thrust. For inclined flight (particularly during the start), most thrust will be supplied by engines to have additional positive moment about COG. This should leads the airship to elevate a nose.

In case of airship ascent or descent, direction of motion is not symmetrical with hull centre line, but it seize an angle between these two lines. This effect causes asymmetrical  pressure distribution over the shell and creates additional dynamic force. For an example of nose elevated airship moving forward, due the increased pressure below the hull, center of pressure over the shell lies down the hull center line and causes force acting in positive direction of rotation, adding the positive moment about COG, so this force trends to elevating the nose up. This force, called dynamic lift of hull, is hard to determined numerically because the direction of motion angle is not easily predictable in decency of hull center line angle. In airship design is described with empirical formula based on real model measurements. For this design purpose, this force is omitted and it is assumed that hull centerline angle and direction of motion is the same. Therefore pressure distribution is symmetrical over the shell and resultant drag force lies directly on center line.

Calculation example for inclined flight:

traveling speed

thrust ;

rudder setup   

From fluid dynamic analysis can be determined shell drag force  and tail drag force , .

For unknown center line angle , value can be expressed from static equilibrium as

which is solved for given inputs .

Control

Pitching and yawing rudders have got the same geometry. Kinematic of control mechanism is designed to have the same maximal angle to positive as well to negative rudder deviation. Therefore the controlling has got symmetrical behaviour to both direction. For each rudder, two of parallel acting servo drives is designed so each servo balances a half of force on rudder surface.

Kinematic of control mechanism is described bellow.

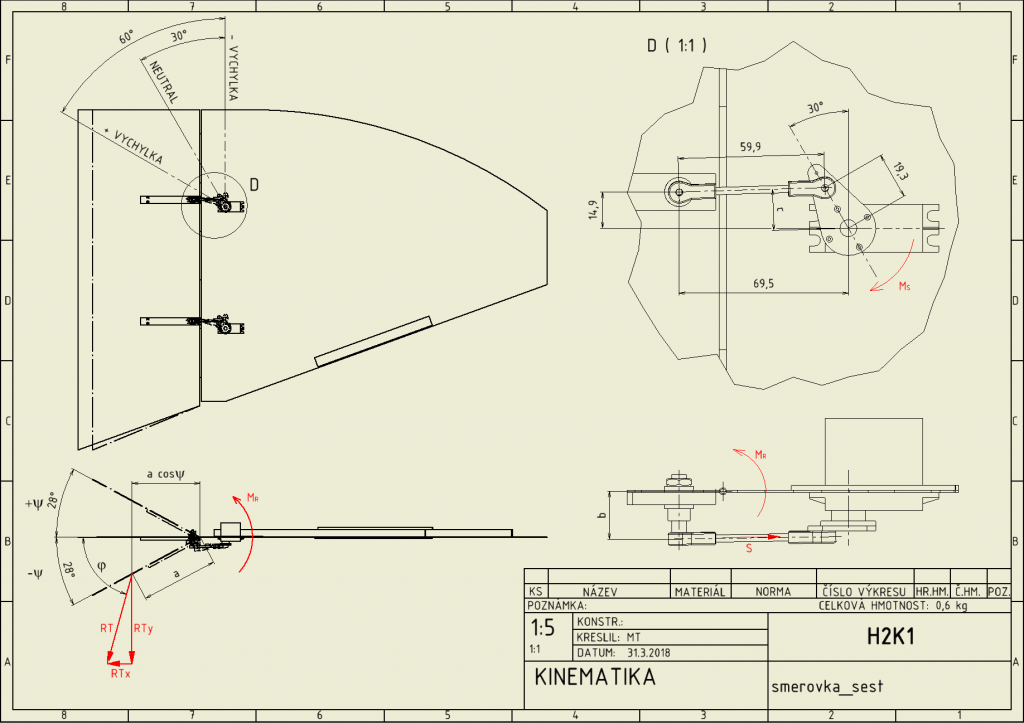
For moment about the axis of rudder rotation can be written static equilibrium

From this equation, force on control rod can be expressed and with substitution to equation for moment about servo axis, moment on servo shaft can be written as

Calculation example of servo moment:

traveling speed

rudder setup   

From fluid dynamic analysis can be determined tail drag force , which gives for one servo;  .

distance  and is for each maximal deviation

                     

                     

For given inputs, moment on servo shaft is

          

For chosen servo type, maximal torque is .Based on this calculations, the servo drives are able to operate with fully deviated rudders up to travel speed safely.

Engines

Propeller type had been chosen on basic of required parameters. Calculation of propeller’s static thrust had been done with program at website: https://rcplanes.online/calc_thrust.htm

With 11.1V battery and engine with 1050 RMP/V, propeller static RMP is 11655 rev/min. For chosen propeller type APC SP 10×3.8,  it is computed estimated static thrust as 2.318kg.

Assumed for horizontal flight with full thrust of , it gives .

With comparison of shell drag force characteristic, for full thrust of upper engines, theoretical travel speed is approx. .

Motor maximal peak current gives motor input power .

 

Design conclusion

Airship is designed for safety operation up to nominal travel speed . Anyway based on calculations, it is assumed that airship maximal speed should reach approx. .

With shell volume and gross lift force    and with total weight  , remains for free lift, which should be enough for omitted parts weights (RC accessories, rivets, electronics,…)

Most of useful information about airship aerodynamic had been drawn in publication of US WAR DEPARTMENT technical manual of 1941, which can be found at the link https://www.faa.gov/regulations_policies/handbooks_manuals/aviation/media/airship_aerodynamics.pdf.

With next step, working drawing will be processed in detail, and realization will continue.